HERBERT W. FRANKE
Herbert Werner Franke, born in Vienna on May 14, 1927, studied Physics and Philosophy there. In 1951 he received his Doctorate in Theoretical Physics. Today Franke is known as the most important post-war German-language writer in the genre of science fiction. Franke is also recognised worldwide as a pioneer of algorithmic art. His intellectual work is based equally on the rationality of the researcher and the creativity of the artist. He is particularly interested in creating aesthetically interesting structures with the help of computer programs. In addition to creating works of art, Franke has also been intensively involved in questions of rational aesthetics. In his "Rational Theory of Art," published as early as the mid-1960s, he described the perception of art as a construct that can be grasped with the help of information theory.
Direct Link to the store to browse and purchase all available works HERE.
MATH GOES ART - A SOLO EXHIBITION BY H.W. FRANKE
(from 21st July -31st October, 2021 feat. unique vintage works, which were never exhibited before).
This page highlights some details about the current exhibition, we are glad to introduce 3 main body of works within Herbert W. Franke concept of Math Art where the artist has been exploring and researching the visual side of mathematics : Drakula(1970s), Cellular Automata (1990s), Lissajous (1998). We are offering you the chance to preview some of the works from each period and reserve one by clicking the link below each section.
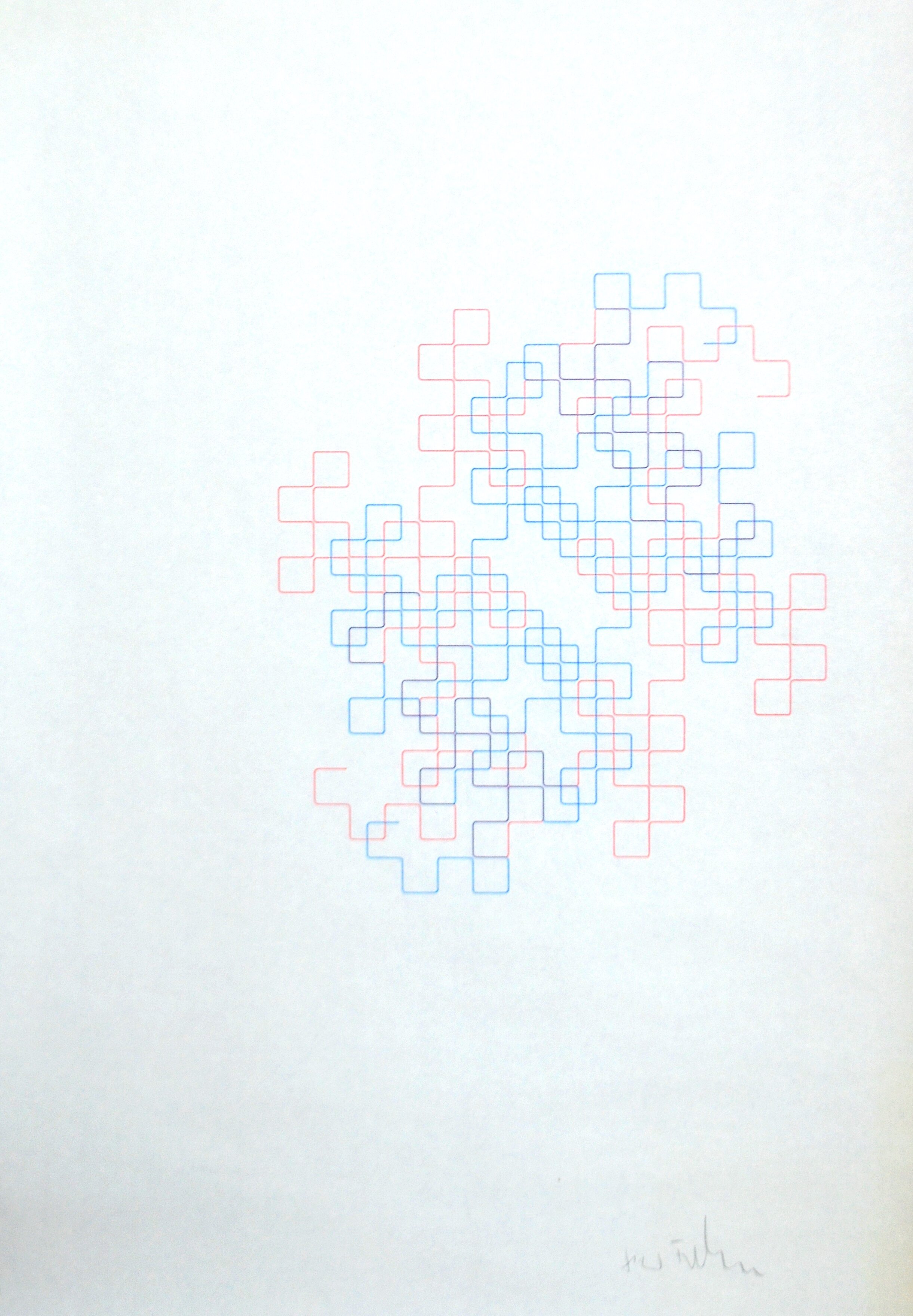
Drakula (1970/71)
The dragon curve is a fractal object. It was first studied by NASA physicists Johnm Heighway, Bruce Banks and William Harter and described by Martin Gardner in his 1967 column Mathematical Games in Scientific American. However, the basic mathematical theory, was not developed until 1970 by mathematician Chandler Davis and computer scientist Donald Knuth. For Franke, the dragon curves were an ideal instrument as a study object of experimental aesthetics, since they could be quantified well in terms of information theory. Dragon curves are formed by sequences of left and right turns according to certain rules. List of all works from these series.
Cellular Automata (since 1992)
For many sciences, it is advisable to study the simplest cases first. This is also possible for abstract world models. One way to do this is through game theory. In search of the simplest possibilities, Stephen Wolfram came up with the so-called one-dimensional cellular automata. The underlying world consists of a distribution of elements along a straight line. Each element is assigned a variable index. This changes in the time by a fixed "world law", which prescribes whole-numbered changes of the ratios. If one brings this law like expiry in "time quanta” these ratios change according to the postulated regularities. The development running in the time - in "time quanta" representable -, can be shown by the change of the key figures from one line to the next line. The result is shown as a two-dimensional process image, which is also aesthetically remarkable. If one now describes the key figures of the “time quanta” by colors and puts these lines directly one below the other, the historically growing picture of a world is built up on the basis of the given laws. It comes in dependence of the given laws to structure formations.
6 vintage unique prints pieces, format (paper outside) 59 x 42 cm - format image 54 x 29,5 cm.
Lissajous figures (since 1998)
Lissajous figures are curve graphs that result from the superposition of two oscillations. They are named after the French physicist Jules Antoine Lissajous (1822-1880). The programme of the series with Lissajous figures belongs to the so-called Math Art, with which Herbert W. Franke visualized different mathematical principles, equations and theories. If you are interested in any of Lissajous digital works, please contact us here.
6 analogue graphics, 1956/57
Vintage ferrotype gelatin silver print on baryta paper, all framed
Back in the 2019 we had the pleasure to feature Herbert W. Franke precious Oscillograms from 1956/57’ as part of our extensive exhibition ‘Automat und Mensch’ on the history of AI and generative art.
Interview Part I in German.
Interview Part II in German.
Exclusive English versions available in the edition XII of the gallery’s quarterly magazine ‘Collecting digital art’.
All images above are under a copyright by Herbert W. Franke : Archive space press. Unauthorized reproduction is prohibited.
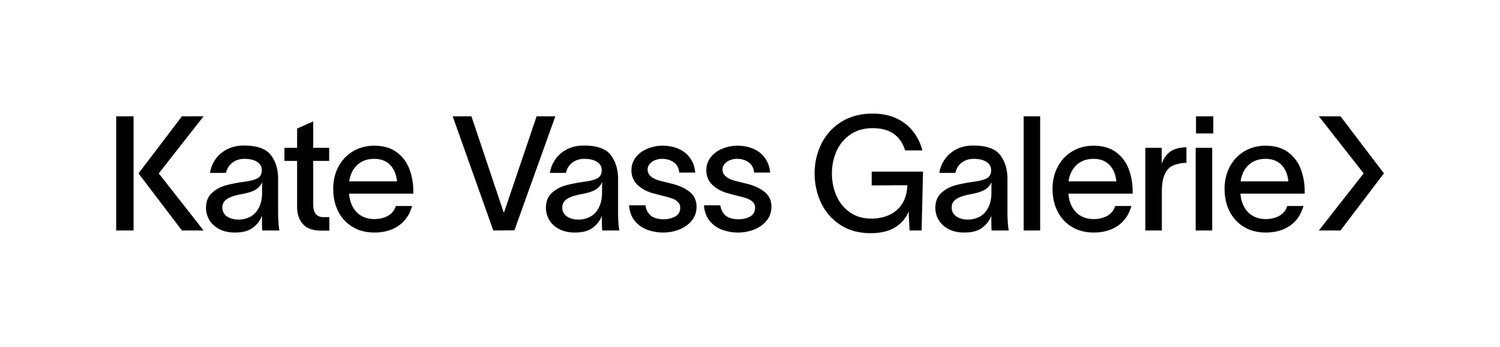
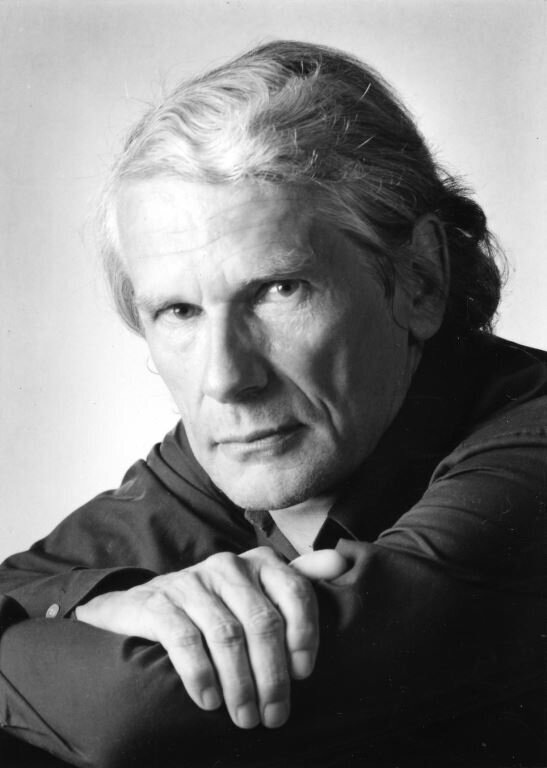
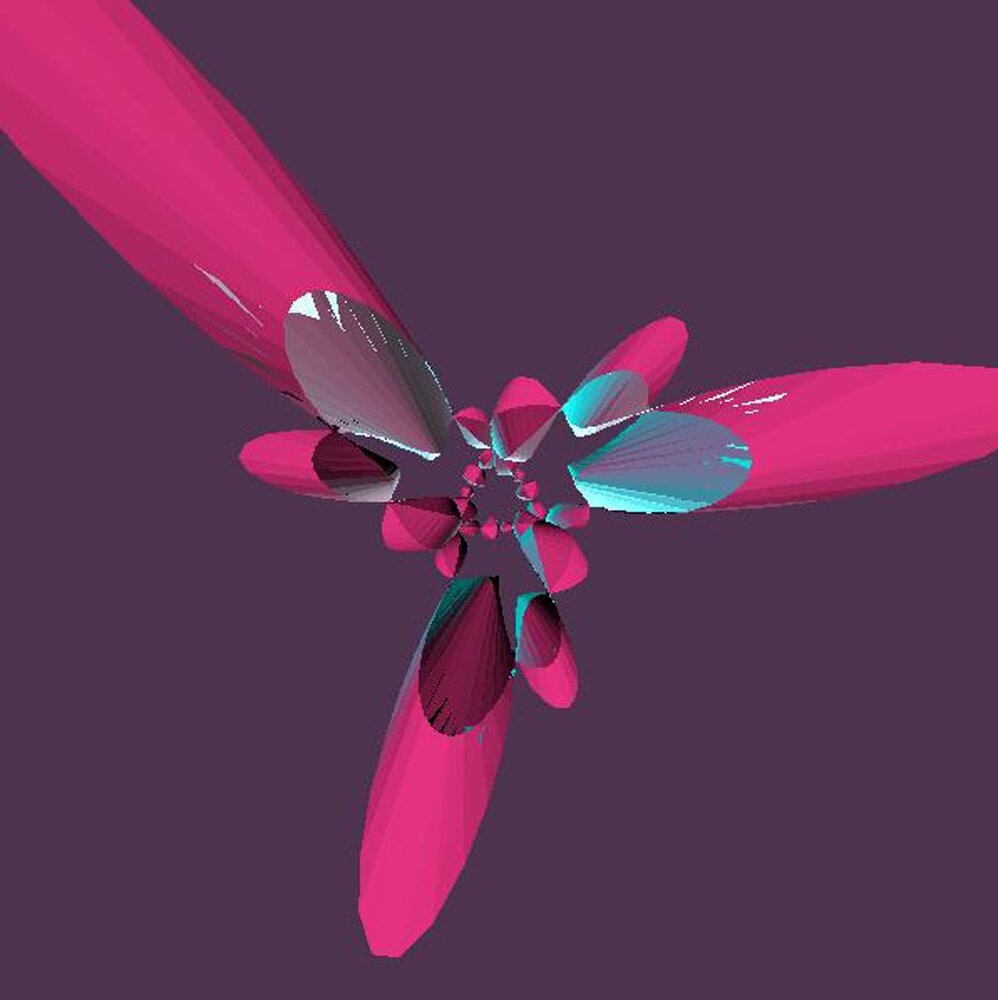
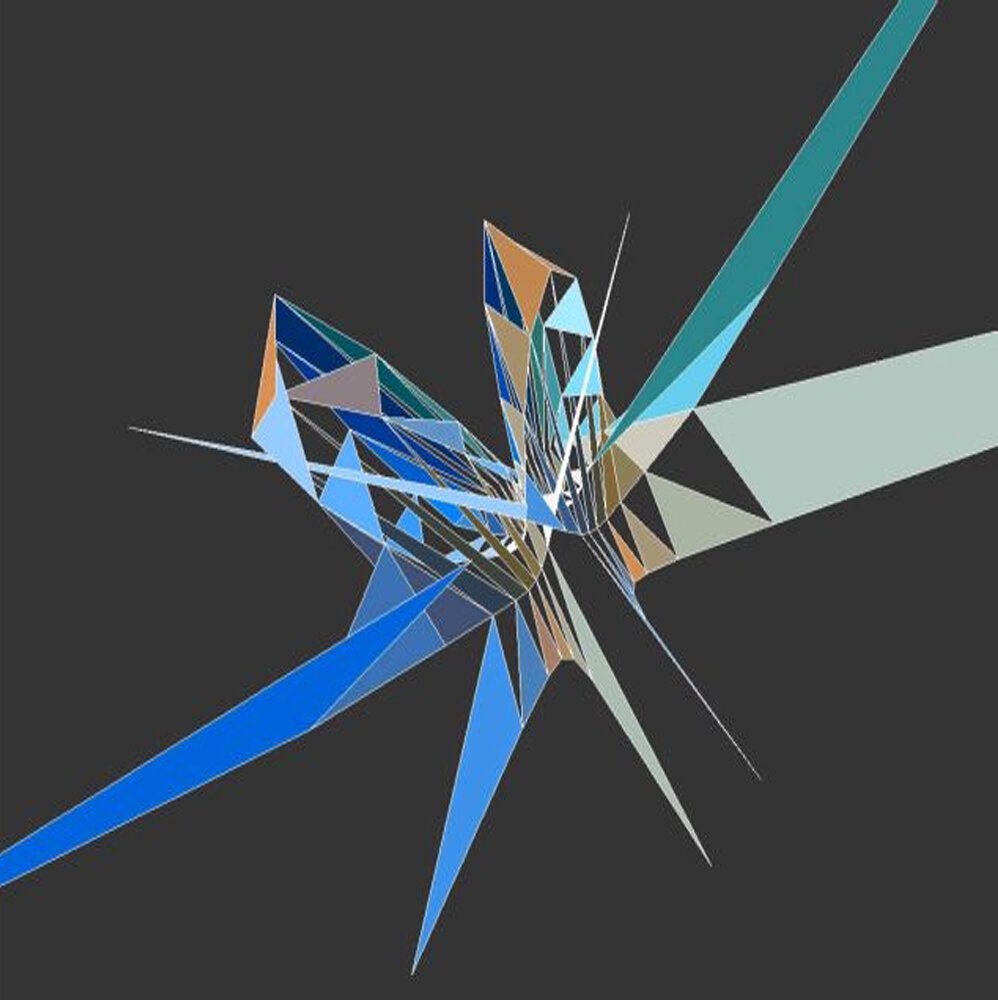
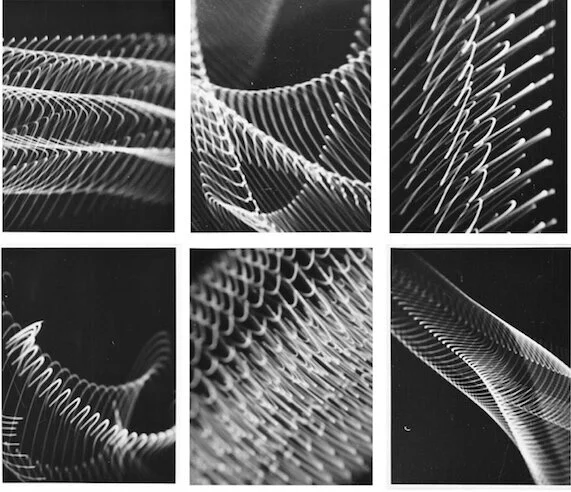
Oscillogram, 9 analogue graphics, 1956/57
Vintage ferrotyped gelatin silver print on baryta paper
Size: each 24 x 18 cm
Framed
Exhibited at “Automat und Mensch” show, Zürich, Kate Vass Galerie, 2019