MATH GOES ART
a solo show by Herbert W. Franke - a pioneer of computer art.
July 21st, 2021 - 31st October 2021
The Heighway dragon. The Dragon curve was discovered (or shall one say, invented) by a NASA physicist John Heighway in 1966 and named by his colleague William Harter.
The emergence of art is associated with man's desire to learn about the world around him. Art became a powerful tool in the struggle for existence. It helped to create a model of the world, to study it and identify relationships. As human developed, he took art to a new level, which promoted a man to a new development stage.
One can argue that art's primary function was to make it easy to present and analyze information, with the possibility of identifying patterns. That is the kind of task mathematics was designed to perform. In other words, a characteristic feature of both art and mathematics is that they strive to develop and transcend the dominant norm achieved. The question of beauty has inspired many artists, scientists and philosophers for centuries. Today's study of aesthetics is an active research area in fields as diverse as psychology, neuroscience, and computer science. Herbert W. Franke has been interested in mathematical aesthetics for the last decades and had experimented with algorithms and computer programs to visualize math in art.
"Art is always about mathematics. Every image can be described mathematically. This also applies to music, take, for example, the theory of harmony in music. There are examples from the history of art - the so-called `golden ratio`, which has its origins in ancient Greece and has always been considered the essence of aesthetics. There is nothing more than a formula behind it. Human perception is a process of data processing. We analyze, filter and encode all information that affects us. Above all, filtering is crucial because we have only limited processing capabilities." states Franke.
Herbert W. Franke proposed a theory based on psychological experiments that suggested that working memory can't take more than 16 bits per second of visual information. Then he argued that artists should produce a flow of information of about 16 bits per second for their works of art to be perceived as beautiful and harmonious.
“Psychological tests have shown that we perceive something optimally when information enters our consciousness at a rate of about 16 bits per second. In colloquial language, this would be described as 'beautiful' or 'harmonic'. This behaviour also applies to the perception of art.”
One can argue that art is always the result of creative genius, which nevertheless obeys natural laws. "As living beings, we follow principles of order that are common to all human beings. For example, symmetry: All higher living beings have symmetrical anatomy. Symmetry helps us to orient ourselves more quickly. It is based on mathematical rules, just like the principle of continuity. Why are we able to catch a ball when it is flying towards us? Because we can predict its trajectory: The "continuous trajectory" is based on the laws of classical physics. For humans, it expresses harmony because it is a matter of undisturbed movements. This is also the reason why we perceive movements in sports or dance as harmonious. The same is true for pictures. The artist produces such steady curves involuntarily and without technical aids" - argues Franke.
While the painter uses the brush intuitively, Herbert W. Franke consciously relies on calculations. In both cases, a visual offer of information is created that the viewer can deal with through perception.
But if all art is derived from mathematical formulas, then where is artistic creativity left? - you may ask. Creativity is the ability to bring forth something new. Chance plays an essential role in this because it brings forth the unpredictable, i.e. something new in principle. Modern quantum physics show us that there are events for which we do not recognize any causal connection. In the creative artistic process, however, the chance is the prerequisite for complexity and thus for his works' structuring. On the other hand, art does without structure and only wants to trigger feelings through associations, does not require the viewer or listener to engage with the work of art, but only the emotional effect. It lies below our capacity to absorb information.
The father of computer art, one of the leading German science fiction authors - Professor Herbert W. Franke, got fascinated about imaging systems early at the end of the 1940s when he was given the task of calculating electric-magnetic fields, as used in electron microscopes. He comments: "it opened my eyes to things I had never seen before, such as beautiful crystalline landscapes. It soon became clear that if the electric fields were deliberately calculated differently, quite adventurous figures would emerge. That gave me the idea of using other tools of technical laboratories, such as oscillographs and later computers, to create images for artistic purposes."
Current exhibition focuses on three main body of works within Herbert W. Franke concept of Math Art where the artist has been exploring and researching the visual side of mathematics : Drakula(1970s), Cellular Automata (1990s), Lissajous (1998).
Drakula series (1970/71)
Dracula Series from seventies was an ideal instrument as a study object of experimental aesthetics, since they could be quantified well in terms of information theory. The Heighway dragon curves (aka the Harter–Heighway dragon or the Jurassic Park dragon) was first discovered by NASA physicists John Heighway, Bruce Banks, and William Harter. It was described by Martin Gardner in his Scientific American column Mathematical Games in 1967, where he stated that Harter used the dragon as a cover design for a booklet prepared for a NASA seminar on group theory. However, the basic mathematical theory was not created until 1970 by the mathematician Chandler Davis and the computer scientist Donald Knuth.
The Heighway dragon can be constructed from a base line segment by repeatedly replacing each segment by two segments with a right angle and with a rotation of 45° alternatively to the right and to the left.
Franke's 'dragon curves' are formed by sequences of left and right turns according to certain rules. The program allows to string together and superimpose dragon curves of different orders - or even sections of such curves. Left and right turns were not only represented in the usual way by right-angled bends, but by mathematically defined elements, triangles or multiple curved curve sections. These elements were designed in such a way that when they are superimposed, clearly recognizable new form elements are created by overlaps and appositions. The superimposition of closed curves in different colors is particularly attractive.
Unique Drakula Vintage works as well vintage edition prints are available, signed by artist.
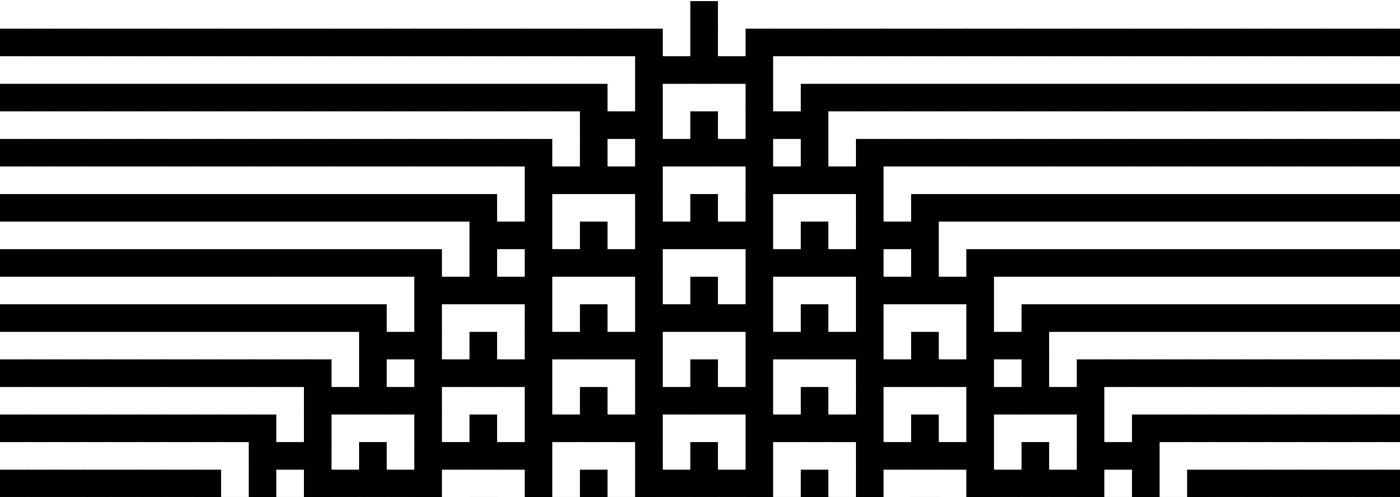
Heighway dragon. The version that Harter used for the NASA cover, and which was reproduced in Gardner's column, looked like the following (with 11 iterations, but the original had rounded corners.)
Cellular Automata (since 1992)
Converting mathematics into images does not only helps a better understanding of equations and formulae, but also leads straight into art. For many sciences, it is known to study the simplest cases first. This is also possible for abstract world models. One way to do this is through famous game theory. In search of the simplest possibilities, Stephen Wolfram came up with the so-called one-dimensional cellular automata. The underlying world consists of a distribution of elements along a straight line. Each element is assigned a variable index. This changes in the time by a fixed "world law", which prescribes whole-numbered changes of the ratios. If one brings this law like expiry in "time quanta” these ratios change according to the postulated regularities. The development running in the time - in "time quanta" representable -, can be shown by the change of the key figures from one line to the next line. The result is shown as a two-dimensional process image, which is also aesthetically remarkable. If one now describes the key figures of the “time quanta” by colors and puts these lines directly one below the other, the historically growing picture of a world is built up on the basis of the given laws. It comes in dependence of the given laws to structure formations. Herbert W. Franke has experimented with numerous visualizations of such dynamically growing world models, analyzing in particular the influence of random generators on their development and also used them for artistic works. The outcomes you can experience in his series of works ‘Cellular Automata’ please see the great selection of videos and vintage unique prints, signed by the artist.
The videos show Visualizations of Cellular Automata
Lissajous figures (since 1998)
Shape of Lissajous figures for various ratios of periods. In the pre-digitization era, mathematicians and scientists were very clever in using what was available to investigate the methods of natural phenomena at the time. Among the most common techniques was the use of small mirrors suspended from threads, tuning forks, or springs because the harmonic motion of the placement was sinusoidal. They traced the waveform with a beam of light (not a laser, of course) directed at a mirror and a reflection directed at a flat surface.
The last chapter covers the later period of Herbert Franke so-called Math Art, with which he visualized different mathematical principles, equations and theories. Lissajous figures combine mathematical elegance, engineering applications, and artistic possibilities. Lissajous figures (or curves): Even if you don’t know what the name refers to, you’ve most probably seen them. Lissajous figures can be defined as any of an infinite variety of curves formed by combining two mutually perpendicular simple harmonic motions, commonly exhibited by the oscilloscope, and used in studying frequency, amplitude, and phase relations of harmonic variables. They are named after the French physicist Jules Antoine Lissajous (1822-1880). Lissajous curves are sometimes also known as Bowditch curves after Nathaniel Bowditch, who studied them in 1815, but were studied in more detail by Jules-Antoine in 1857.
Both Lissajous series shown here are 3D, the symmetric form of the original Lissajous was manipulated by stretching and squeezing it.
Lissajous consists of 11 works in total. Only 4 were minted on opensea.io and now only available from the secondary market.
————
Recommended reading:
Artist Bio: https://www.katevassgalerie.com/math-art-by-herbert-w-franke
Store link: https://www.katevassgalerie.com/print?category=Herbert+W.+Franke
Related articles and interviews:
Interview Part I in German
Interview Part II in German
All images above are under copyright by Herbert W. Franke : Archive space press. Unauthorized reproduction is prohibited.

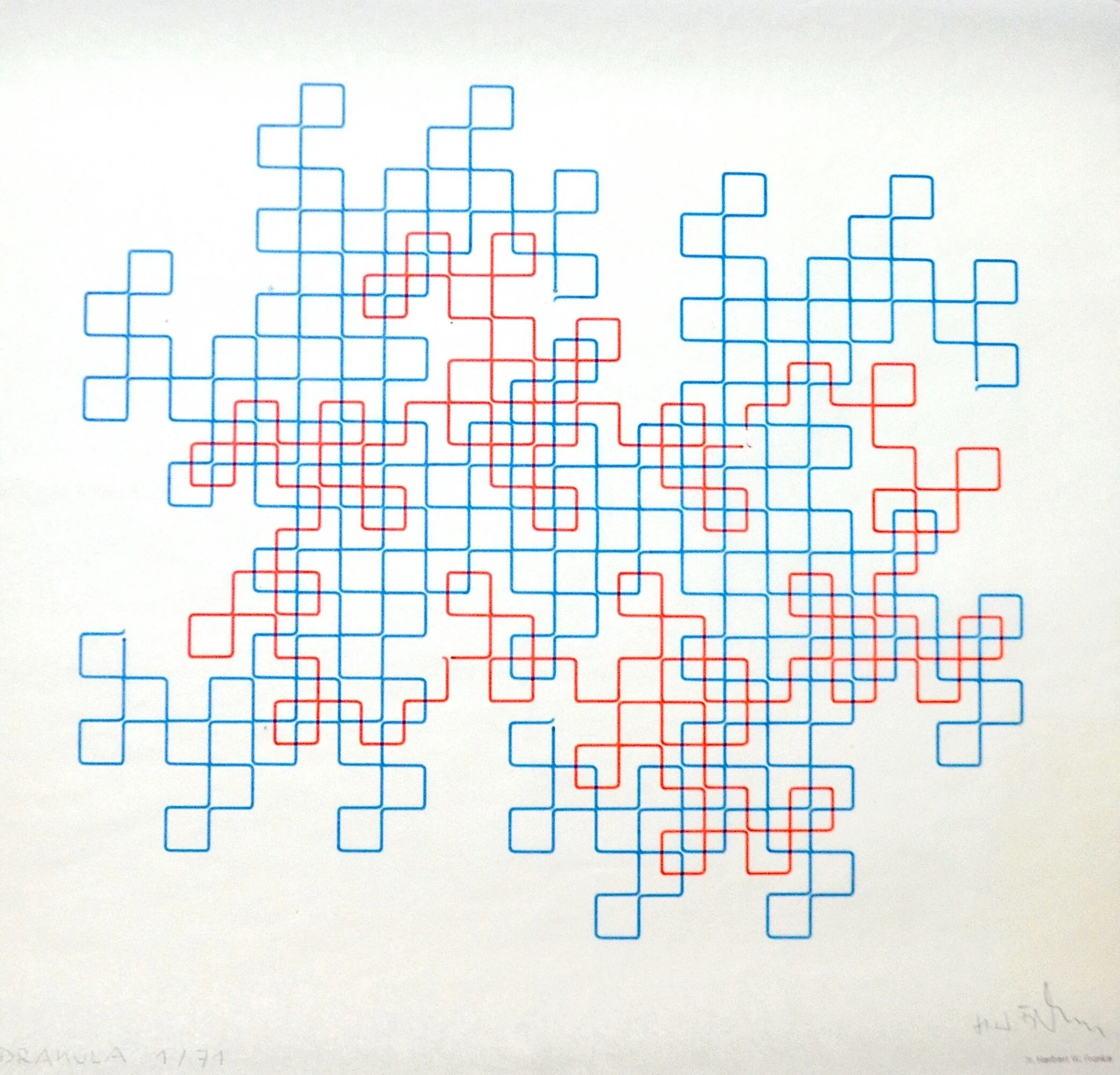



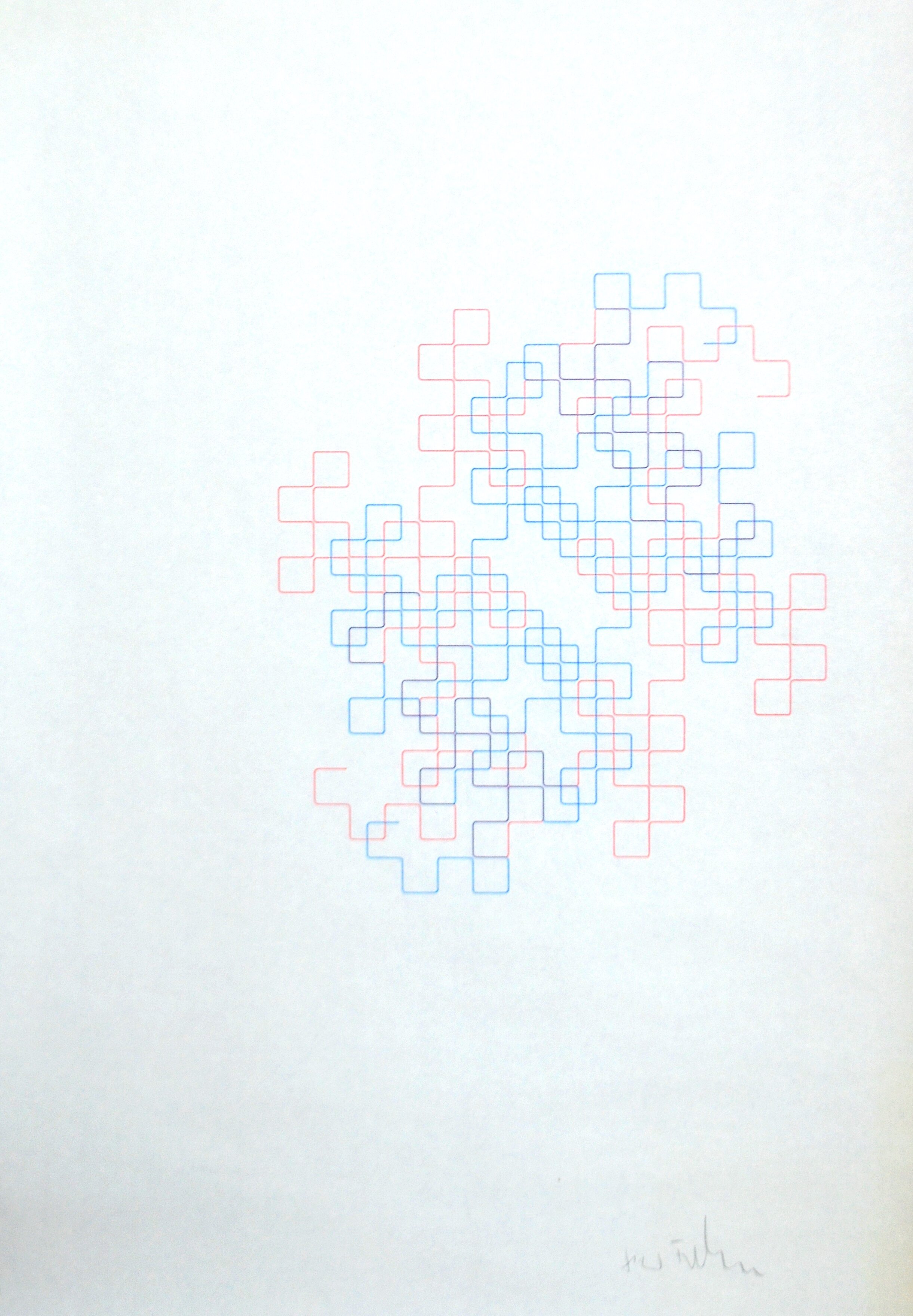











Drakula 22A-71
Limited edition of 15 pieces signed by the artist
TO INQUIRE ABOUT THIS WORK PLEASE CONTACT US: info@katevassgalerie.com
Edition n.2/15
Vintage Print Red and Blue, 1971
Size: DinA4
8.3’x11.7’’inches
21x29.7cm
Paper, Unframed